Answer:
Robert can read the books in 129,600 different ways.
Explanation:
The order in which the book are read is important, which means that the permutations formula is used to solve this question.
Permutations formula:
The number of possible permutations of x elements from a set of n elements is given by the following formula:

Top shelf:
4 books from a set of 6. So

Middle shelf:
2 books from a set of 3. So
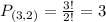
Bottom shelf:
3 books from a set of 6. So
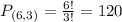
Total:
360*3*120 = 129,600
Robert can read the books in 129,600 different ways.