Question

Function 2

+ 4x + 1
Function _
has the larger maximum.
(Put 1 or 2 in the blank space)

Answer 1

Function 2 has larger max value.

Explanation:

We know that the graph of Function 1 has the maximum value of 1 at x = 4 by looking at the graph.

But since we don't know what the maximum value of Function 2 is (Because Function 2 isn't given as a graph but rather an equation.) which means that we have to find the maximum value of Function 2.

`\large{f(x) = - {x}^(2) + 4x + 1}`

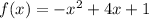
It is not necessary to find x-value for a function because we want to know which function has larger maximum value. We will be using the formula below.

`\large \boxed{y = \frac{4ac - {b}^(2) }{4a} }`

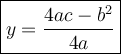
As you may know, a max-min value is indeed y-value. From Function 2, we know the value of a, b and c from standard form y = ax²+bx+c

Substitute our a, b and c in the formula.

`\large{y = \frac{4( - 1)(1) - {(4)}^(2) }{4( - 1)} } \\ \large{y = ( - 4 - 16)/( - 4) } \\ \large{y = ( - 20)/( - 4) = 5}`

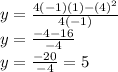
Since our maximum value or y-value is 5 for Function 2. Since we also know that Function 1 has 1 as maximum value and Function 2 has 5 as maximum value. Therefore, Function 2 has larger maximum value.