Answer:
Area of trapezoid = 67.6 square units
Explanation:
Area of a trapezoid is given by the expression,
Area of the trapezoid =
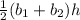
Here,
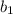 and
and
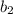 are the parallel sides of the given trapezoid.
are the parallel sides of the given trapezoid.
And '
 ' = Height between the parallel sides
' = Height between the parallel sides
From the given triangle ABE,
m(∠ABE) = m(∠ABC) - m(∠EBC)
m(∠ABE) = 120° - 90°
= 30°
By applying cosine rule in the given triangle,
cos(30°) =
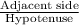
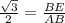
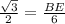
BE =
 units
units
By applying sine rule in ΔABE,
sin(30°) =
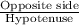
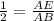
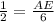
AE = 3 units
Length of

Length of
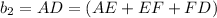 [AE = FD, since given trapezoid ABCD is an isosceles trapezoid]
[AE = FD, since given trapezoid ABCD is an isosceles trapezoid]
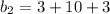

Height between the parallel sides

Area of the trapezoid =
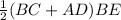
=
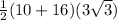
=
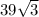
= 67.6 square units