Question

Solve the triangle. Round to the nearest tenth.
39°
16
84°
B

Answer 1

A. m<C = 57 degrees, a = 12, b = 19

Explanation:

First we can start by calculating Angle C by using the rule that all interior angles of a triangle always add to 180 degrees:

180 - (39+84) = C

180 - 123 = C

C = 57

Now let's calculate side a. We can do this by using the sin rule:
`(sin A)/(a)=(sin C)/(c)`

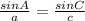
According to the diagram we know that Angle A is 39 degrees, Angle C is 57 degrees, and side c is 16 so we can substitute these values into the formula and solve:

`(sin39)/(a) =(sin 57)/(16) \\\\(0.629320)/(a) =(0.838671)/(16)\\\\0.629320=0.052417a\\0.629320/0.052417=a\\a= 12`

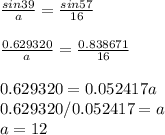
We can use the same method to solve for side b:

`(sin84)/(b) =0.052417 \\0.994522=0.052417b\\0.994522/0.052417=b\\b= 19`

We now have the following values:

Angle C = 57 degrees, a = 12, b = 19

We can now see that A/Number 1 is the correct option.

Hope this helped!