Question

What is the area of rectangle ABCD, if points A, B, C, and D have the following coordinates?


`* A(2, 5), B(2 (2)/(3) , 5) C(2 (2)/(3) , 5 (3)/(5) ), D(2, 5 (3)/(5) )`

Answer 1

The area of the rectangle ABCD is
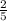`(2)/(5)` square units.

Explanation:

From statement we have that rectangle ABCD is formed by the following points:
`A(x,y) = (2, 5), B(x,y) = \left((8)/(3), 5 \right), C(x,y) = \left((8)/(3), (28)/(5) \right), D(x,y) = \left(2, (28)/(5) \right)`. First, we calculate the length of each side by the Pythagorean Theorem:

`AB = \sqrt{\left((8)/(3)-2 \right)^(2)+ (5-5)^(2)}`

`AB = (2)/(3)`

`BC = \sqrt{\left((8)/(3)-(8)/(3)\right)^(2)+\left((28)/(5)-5\right)^(2) }`

`BC = (3)/(5)`

`CD = \sqrt{\left(2-(8)/(3) \right)^(2)+\left((28)/(5)-(28)/(5) \right)^(2)}`

`CD = (2)/(3)`

`DA = \sqrt{\left(2-2\right)^(2)+\left(5-(28)/(5) \right)^(2)}`

`BC = (3)/(5)`

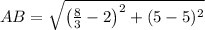
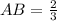
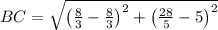
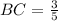
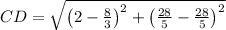
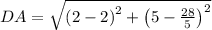
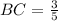
Which satisfies all minimum characteristics for a rectangle. The area of the rectangle ABCD is the product of its base and its height, that is:

`A = \left((2)/(3)\right)\cdot \left((3)/(5) \right)`

`A = (2)/(5)`

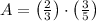
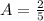
The area of the rectangle ABCD is
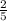`(2)/(5)` square units.