Question

Factorise y^6 - 64 as possible
​

Answer 1

(y - 2)(y + 2)(
`y^(4)` + 4y + 16)

Explanation:

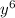`y^(6)` - 64 ← is a difference of cubes and factors in general as

a³ - b³ = (a - b)(a² + ab + b²) , then

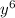`y^(6)` - 64

= (y² )³ - 4³

= (y² - 4)(
`y^(4)` + 4y + 16) ← note y² - 4 is a difference of squares

= (y - 2)(y + 2)(
`y^(4)` + 4y + 16)

Answer 2

Explanation:

Observe that
`y^6-64=y^6-2^6.`

And you factor out using this expression:

`(a^6-b^6)=(a-b)(a^5+a^4b+a^3b^2+a^2b^3+ab^4+b^5)`

Take a=y and b=2.