Question

Steve ran a 26-mile race at an average speed of 4 miles per hour. If Adam ran the same race at an average speed of 3 miles per hour, how many minutes longer did Adam take to complete the race than did Steve?

Answer 1

Adam took 132 minutes longer than Steve.

Explanation:

Let's find the time that takes Steve and Adam to complete the race. We will use the equation:

`V = (d)/(t)`

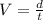
Where:

d: is the distance = 26 miles

t: is the time =?

V: is the speed

For Steve we have the following time:

`t_(s) = (d)/(V_(s)) = (26 mi)/(4 mi/h) = 6.5 h*(60 min)/(1 h) = 390 min`

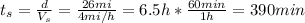
And the time of Adam is:

`t_(a) = (d)/(V_(a)) = (26 mi)/(3 mi/h) = 8.7 h*(60 min)/(1 h) = 522 min`

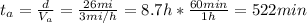
So, the difference between the time of Adam and Steve is:

`\Delta t = t_(a) - t_(s) = 522 min - 390 min = 132 min`

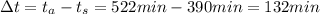
Hence, Adam took 132 minutes longer than Steve.

I hope it helps you!