Question

How many blocks with dimensions of One-third times 1 times 1 can fit in a unit cube?

A block has a length of one-third, height of 1, and width of 1.
2
3
6
9

Answer 1

the answer would be "b" 3 because its in 1/3 and the block is 1/3

Answer 2

Given:

Dimensions of a block are
`(1)/(3)* 1* 1`.

To find:

The number of block that can be fit in a unit cube.

Solution:

Volume of a cuboid is:

`V=l* b* h`

Where, l is length, b is breadth or width and h is the height of the cuboid.

So, the volume of the given block is:

`V_1=(1)/(3)* 1* 1`

`V_1=(1)/(3)`

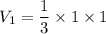
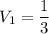
Dimensions of a unit cube are
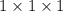`1* 1* 1`. So, the volume of the unit cube is:

`V_2=1* 1* 1`

`V_2=1`

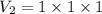
We need to divide the volume of unit cube by the volume of a block to find the number of block that can be fit in a unit cube.

So, the number of blocks that fit in a unit cube is:

`n=(V_2)/(V_1)`

`n=(1)/((1)/(3))`

`n=3`

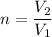
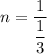
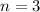
Therefore, the correct option is B.