Answer:
Following are the answer to the given question:
Explanation:
Following are the null and alternative Hypothesis:
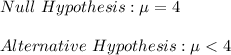
Rejection Zone
This is left tailed test, for α = 0.2 and df = 39
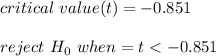
Testing statistic:

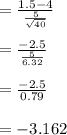
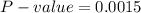
P-value < 0.2, reject the null hypothesis.
Approach to the Rejection Zone:
The null hypothesis is rejected since the test statistic t is outside of the critical range value. Only at the 0.200 significance mark, there's significant evidence that its new shrub's growth rate is less than 4 cm per week.