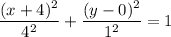Question

The center of an ellipse is (-4,0) one vertex is (-8,0) and one co-vertex is (-4,1). Write the equation of the ellipse

Answer 1

The equation of the ellipse is;

`((x + 4)^2)/(4^2) + ((y - 0)^2)/(1^2) = 1`

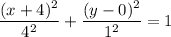
Explanation:

The parameters of the ellipse are;

The location of the center of the ellipse = (-4 0)

The coordinates of the vertex of the ellipse = (-8, 0)

The coordinates of the the co-vertex = (-4, 1)

The general form of the equation of an ellipse is presented as follows;

`((x - h)^2)/(a^2) + ((y - k)^2)/(b^2) = 1`

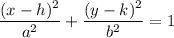
The center of the above equation of an ellipse = (h, k)

The vertex of the above equation are; (h - a, k) and (h + a, k)

The co vertex of the above equation are; (h, k - b) and (h, k + b)

By comparison, we have;

h = -4, k = 0

For the vertex, we have;

When

h - a = -8

∴ -4 - a = -8

-a = -8 + 4 = -4

a = 4

When

h + a = -8

-4 + a = -8

∴ a = -4

a = 4 or -4

We note that a² = 4² = (-4)²

For the covertex, we have;

When

k - b = 1

0 - b = 1

b = -1

When k + b = 1

0 + b = 1

b = 1

∴ b = 1 or -1

b² = 1² = (-1)²

We can therefore write the equation of the ellipse as follows;

`((x - (-4))^2)/(4^2) + ((y - 0)^2)/(1^2) = ((x + 4)^2)/(4^2) + ((y - 0)^2)/(1^2) = 1`

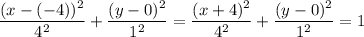
Therefore;

`((x + 4)^2)/(4^2) + ((y - 0)^2)/(1^2) = 1`