Answer:
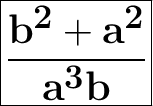
Explanation:
Given expression:
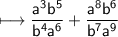
Solution:
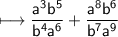
Cancel a^3 and a^6 on the left hand side of plus sign, which results to a^3.
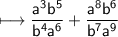
That is,
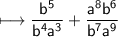
Cancel b^5 and 5^4 on the LHS of plus sign, which results to b.

That is,
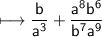
Now cancel a^8 and a^9 on the RHS of Plus sign, which results to a.
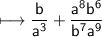
That is,
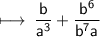
Cancel b^6 and b^7 on the RHS of the Plus sign, which results to b.
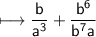
That is,
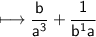
Simply add:-
Rewrite into:
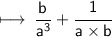
Combine the numerators over LCD(a^3)
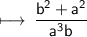
Or it can also be rewritten as,
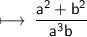
________________________________
I hope this helps!
Please let me know if you have any questions