Answer:
The pvalue of the test is 0.7188 > 0.05, which means that there is not enough statistical evidence based on the sample to dispute the results of the study published in Psychiatry Research.
Explanation:
Test if there is enough statistical evidence based on the sample to dispute the results of the study published in Psychiatry Research:
This means that at the null hypothesis we test if the proportion is 62%, that is:
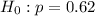
At the alternate hypothesis, we test if the proportion is different from 0.62, that is:
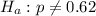
The test statistic is:

In which X is the sample mean,
 is the value tested at the null hypothesis,
is the value tested at the null hypothesis,
 is the standard deviation and n is the size of the sample
is the standard deviation and n is the size of the sample
62% is tested at the null hypothesis:
This means that

When a random sample of 26 autistic children were observed, 17 were found to be left-handed.
This means that

Value of the test statistic:

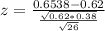

Pvalue of test and decision:
The pvalue of the test is the probability of a proportion that differs from the mean by at least 0.6538 - 0.62 = 0.0338, which is P(|z| > 0.36), which is two multiplied by the pvalue of z = -0.36
Looking at the z-table, z = -0.36 has a pvalue of 0.3594
2*0.3594 = 0.7188
The pvalue of the test is 0.7188 > 0.05, which means that there is not enough statistical evidence based on the sample to dispute the results of the study published in Psychiatry Research.