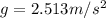Answer:
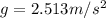
Step-by-step explanation:
From the question we are told that:
Mass Taken
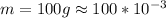
Strain on Spring

Distance pulled down
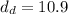
Time for ten oscillations take
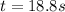
Generally the equation for angular velocity is mathematically given by

Therefore

Generally the equation for Acceleration due to gravity is mathematically given by