Question

(6, - 3, 1) and (8,9,-11) find the angle between the vector​

Answer 1

The angle between the two vectors is 84.813°.

Explanation:

Statement is incomplete. Complete form is presented below:

Let be (6,-3, 1) and (8, 9, -11) vector with same origin. Find the angle between the two vectors.

Let
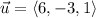`\vec u = \langle 6, -3, 1 \rangle` and
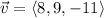`\vec v = \langle 8,9,-11 \rangle`, the angle between the two vectors is determined from definition of dot product:

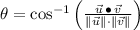`\theta = \cos^(-1) \left((\vec u \,\bullet \,\vec v)/(\|\vec u\|\cdot \|\vec v\|) \right)` (1)

Where:

`\vec u`,
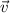`\vec v` - Vectors.

`\|\vec u\|`,
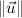`\|\vec v\|` - Norms of each vector.

Note: The norm of a vector in rectangular form can be determined by either the Pythagorean Theorem or definition of Dot Product.

If we know that
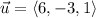`\vec u = \langle 6,-3,1 \rangle` and
`\vec v = \langle 8, 9,-11 \rangle`, then the angle between the two vectors is:

`\theta = \cos^(-1)\left[\frac{(6)\cdot (8) + (-3)\cdot (9) + (1)\cdot (-11)}{\sqrt{6^(2)+(-3)^(2)+1^(2)}\cdot \sqrt{8^(2)+9^(2)+(-11)^(2)}} \right]`

`\theta \approx 84.813^(\circ)`

The angle between the two vectors is 84.813°.