Question

Melissa is a crafting machine! She has used this time in quarantine to finesses her skills and has decided to open up a booth at the Groove Street Farmers Market (Monday’s 4-7pm). She does this for fun but before making her next batch of inventory wants to know which products, she should make to maximize her profit. She makes soaps and candles. The soap sells for $18 and the candles sell for $25. The soap requires coconut oil (2 tablespoons), essential oil (5 drops), and soap base (1 per item). The candles require coconut oil (3 tablespoons), essential oil (8 drops), and wax (1 per item). Coconut oil is $12 a jar and contains 112 tablespoons. Essential oils are $50 a container and contains 150 drops. A soap base is 2$ and a wax base is $2.25. Melissa currently has 3 jars of coconut oil, 2.5 bottles of essential oil, 25 soap bases, and 25 wax bases. If Melissa wants to maximize her profit how many soaps and candles should she make for her next both?

Answer 1

The maximum profit of $847.03 occurs when Melissa produces 25 soaps and 25 candles.

Step-by-step explanation:

The linear programming equations forms as follows:

Cost of producing 1 Soap=Cost of Soap Base+Cost of Coconut Oil+Cost of Essential Oil

Cost of Soap base is $2.

Cost of Coconut Oil for one soap is
`\$(2)/(112)*12`.

Cost of Essential Oil for one soap is
`\$(5)/(150)*50`

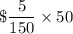
So the total cost of 1 soap is

`\text{Cost of producing 1 Soap}=\$2+\$(2)/(112)*12+\$(5)/(150)*50\\\text{Cost of producing 1 Soap}=\$2+\$0.21428+\$1.6666\\\text{Cost of producing 1 Soap}=\$3.8808`

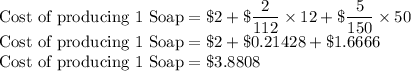
So the cost of producing one bar of soap is 3.8808

So the profit per soap is

`\text{Profit}=\text{Selling Price}-\text{Cost}`

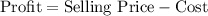
Here selling price is $18 for soap so

`\text{Profit}=\text{Selling Price}-\text{Cost}\\\text{Profit}=\$18-\$3.8808\\\text{Profit}=\$14.1192`

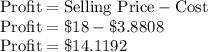
Profit per soap is $14.1192.

Similarly the cost of producing 1 candle is as follows:

Cost of producing 1 Candle=Cost of Wax Base+Cost of Coconut Oil+Cost of Essential Oil

Cost of Wax base is $2.25.

Cost of Coconut Oil for one candle is
`\$(3)/(112)*12`.

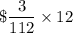Cost of Essential Oil for one candle is
`\$(8)/(150)*50`

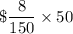
So the total cost of 1 candle is

`\text{Cost of producing 1 Candle}=\$2.25+\$(3)/(112)*12+\$(8)/(150)*50\\\text{Cost of producing 1 Candle}=\$2.25+\$0.32142+\$2.6666\\\text{Cost of producing 1 Candle}=\$5.2380`

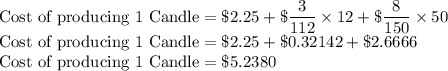
So the cost of producing one candle is $35.2380

So the profit per candle is

`\text{Profit}=\text{Selling Price}-\text{Cost}`

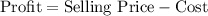
Here selling price is $25 for a candle so

`\text{Profit}=\text{Selling Price}-\text{Cost}\\\text{Profit}=\$25-\$5.2380\\\text{Profit}=\$19.7620`

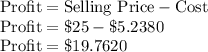
Profit per candle is $19.7620.

If the number of soaps produced is X and the number of candles produced is Y then the maximization function of profit is given as

`Z=f(X,Y)=14.1192X+19.7620Y`

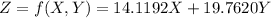
Also the constraints are given as follows:

If Melissa has 3 jars of coconut oil and each jar has 112 tablespoons thus the total tablespoons Melissa has are 336. If 2 tablespoon coconut oil is used for 1 soap and 3 tablespoons are used for 1 candle thus

`2X+3Y\leq336`

Similarly, Melissa has 2.5 containers of essential oil and each container has 150 drops thus the total drops Melissa has are 375. If 5 drops of essential oil are used for 1 soap and 8 drops are used for 1 candle thus

`5X+8Y\leq375`

For the soap bases, each soap uses 1 soap bases and total soap bases are 25 thus

`X\leq25`

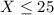
Similarly, for the wax base, each candle uses 1 wax base, and the total wax bases are 25 thus.

`Y\leq25`

So the linear programming model becomes

`2X+3Y\leq336\\5X+8Y\leq375\\X\leq25\\Y\leq25`

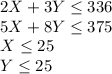
with maximization of

`Z=f(X,Y)=14.1192X+19.7620Y`

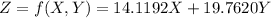
Now solving this using the graphical method of linear programming as attached gives:

The maximum profit of 847.03 occur when Melissa produces 25 soaps and 25 candles.