Given:
A prism has a base area of
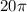 square units and a volume of
square units and a volume of
 cubic units.
cubic units.
To find:
The volume of the cylinder if the cylinder has the same base area and height.
Solution:
Volume of a prism is:
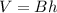
Where, B is the base area and h is the height of the prism.
The volume of cylinder is:
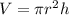
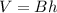
Where, r is the radius, h is the height of the cylinder and
 is the base area.
is the base area.
Since base area and height of the cylinder are same as the prism, therefore, there volumes are equal.
Hence the volume of the cylinder is
 cubic units.
cubic units.