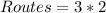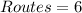Answer:
(a) 36 routes
(b) 6 routes
Explanation:
Given
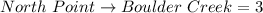
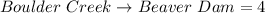
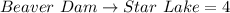
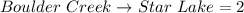
Solving (a): From
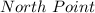 to
to
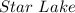 through
through
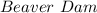
To do this, we consider the following routes;

Taking a pair of routes, we have:
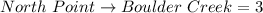
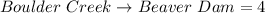
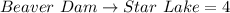
By multiplication route, we have:

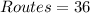
(b) From
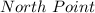 to
to
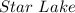 bypass
bypass
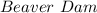 ?
?
To do this, we consider the following routes
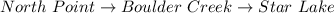
Taking a pair of routes, we have:
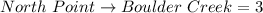
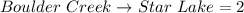
By multiplication rule, we have: