Question

The manufacturer of a certain brand of aluminum foil claims that the amount of foil on each roll follows a Normal distribution with a mean of 250 ft2 and a standard deviation of 2 ft2. To test this claim, a restaurant randomly selects 10 rolls of this aluminum foil and carefully measures the mean area to be ¯ = 249.6 ft2

Find the probability that the sample mean area is 249.6 ft2 or less if the manufacturer’s claim is true

Answer 1

Given that the manufacturers claim is true, the probability that the sample mean area is 249.6 ft.² or less is 0.42074

Explanation:

The manufacturer claims that mean amount of foil on each roll, μ = 250 ft²

The standard deviation of the measured amount of foil per roll, σ = 2 ft.²

The number of rolls tested by the restaurant, n = 10 rolls

The mean measured by the restaurant,
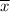`\overline x` = 249.6

Taking the sample means as individual score, we have;

The z-score, of the sample mean is given as follows;

`z=(\overline x -\mu )/(\sigma )`

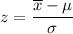
At
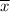`\overline x` = 249.6, we have;

z = (249.6 - 250)/2 = -0.2

Therefore, the probability for a mean less than or equal to 249.6, we have;

The probability, P = P(z < -0.2) = 0.42074

The probability that the sample mean area is 249.6 ft.² or less given that the manufacturers claim is true, P = 0.42074.