Since JK is tangent to circle L, the value of x is equal to 24 units.
In Mathematics and Geometry, Pythagorean theorem is an Euclidean postulate that can be modeled or represented by the following mathematical equation:

Where:
- a is the opposite side of a right-angled triangle.
- b is the adjacent side of a right-angled triangle.
- c is the hypotenuse of a right-angled triangle.
In order to determine the length of JM or the value of x, we would have to apply Pythagorean's theorem as follows;
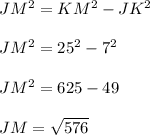
JM = x = 24 units.