Question

Is the following number rational or irrational ? -0. overline 32

Answer 1

The number
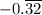`-0.\overline{32}` has a repeating decimal and can be expressed as the fraction -32/99, which means it is a rational number.

Step-by-step explanation:

The number given is
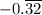`-0.\overline{32}` , which has a repeating decimal pattern. To determine if this number is rational or irrational, we need to establish if it can be expressed as a fraction with integers in the numerator and denominator. A repeating decimal is always considered a rational number because it can be written as a fraction. For example,
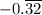`-0.\overline{32}` can be expressed as -32/99, where -32 is the numerator and 99 is the denominator. Therefore,
`-0.\overline{32}` is a rational number.

Answer 2

yes

Step-by-step explanation:

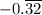because -0 is not rational number