Question

An auto manufacturing company wants to estimate the variance of miles per gallon for its auto model AST72. A random sample of 24 cars of this model showed that the standard deviation of miles per gallon is 0.58. At the alpha = 1%, can the research department conclude that the population variance of miless per gallon for AST72 is decreased from historical value of 0.35?

A. At alpha = 1%, they reject the null hypothesis. The variance of miles per gallon for AST72 model has not decreased from the historical value of 0.35.
B. At alpha = 1%, they failed to reject the null hypothesis. The variance of miles per gallon for AST72 model has not decreased from the historical value of 0.35.
C. At alpha = 1%, they failed to reject the null hypothesis. The variance of miles per gallon for AST72 model has decreased from the historical value of 0.35.
D. At alpha = 1%, they reject the null hypothesis. The variance of miles per gallon for AST72 model has not decreased from the historical value of 0.35.

Answer 1

Since p-value ( 0.4467 ) is greater than significance level ( 0.01 )

Fail to Reject Null hypothesis H₀ at 1%.

At 1% significance level, we can conclude that, population variance is maintained at historic value.

Thus, Option ((B) At alpha = 1%, they failed to reject the null hypothesis. The variance of miles per gallon for AST72 model has not decreased from the historical value of 0.35) is the correct answer.

Explanation:

Given the data in the question;

sample size n = 24

Sample Standard deviation S = 0.58

Let δ represent the population standard deviation

so we will test;

H₀ : δ = √0.35 ≈ 0.5916

H₁ : δ < √0.35 ≈ 0.5916

Test Statistics;

we determine our test statistics as follows;

Z = [√(2(n-1))] × [ lnS - lnδ ] ~ N(0, 1 )

Now, Under H₀,
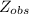`Z_{obs` = [√(2(24-1))] × [ ln (0.58 / 0.5916) ]

= 6.7823 × -0.0198026

= -0.134

From z-table;

p-value = P( z <
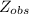`Z_{obs` ) = p( z < -0.134 ) = 0.4467

p-value = 0.4467

Since p-value ( 0.4467 ) is greater than significance level ( 0.01 )

Fail to Reject Null hypothesis H₀ at 1%.

At 1% significance level, we can conclude that, population variance is maintained at historic value.

Thus, Option ((B) At alpha = 1%, they failed to reject the null hypothesis. The variance of miles per gallon for AST72 model has not decreased from the historical value of 0.35) is the correct answer.