Question

There are 320 students in a school. 16 come to school by car. 96 walk to school. Estimate the probability that a particular student: a arrives by car b walks to school c does not walk to school d does not walk or come by car.

Answer 1

a. The probability that a particular student arrives by car is
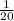`(1)/(20)` = 0.05, which equals 5%.

b. The probability that a particular student walks to school is
`(3)/(10)` = 0.3, which equals 30%.

c. The probability that a particular student does not walk to school is
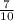`(7)/(10)` = 0.7, which equals 70%.

d. The probability that a particular student does not walk or come by car is
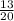`(13)/(20)` = 0.65, which equals 65%.

Explanation:

Probability is the greater or lesser possibility of a certain event occurring. In other words, probability establishes a relationship between the number of favorable events and the total number of possible events. Then, the probability of any event A is defined as the quotient between the number of favorable cases (number of cases in which event A may or may not occur) and the total number of possible cases. This is called Laplace's Law.

`P(A)=(number of favorable cases)/(number of possible cases)`

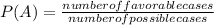
In this case, the number of possible cases is always the same, which is equal to the total number of students. So the number of possible cases is 320 students. The number of favorable cases varies as follows:

a. Number of favorable cases= number of students that arrive by car= 16

So:
`P(A)=(16)/(320)`

P(A)=
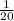`(1)/(20)` = 0.05, which equals 5%

The probability that a particular student arrives by car is
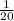`(1)/(20)` = 0.05, which equals 5%.

b. Number of favorable cases= number of students that walk to school= 96

So:
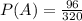
`P(A)=(96)/(320)`

P(A)=
`(3)/(10)` = 0.3, which equals 30%

The probability that a particular student walks to school is
`(3)/(10)` = 0.3, which equals 30%.

c. Number of favorable cases= number of students that do not walk to school = 320 students - number of students that walk to school= 320 students - 96 students= 224 students

So:
`P(A)=(224)/(320)`

P(A)=
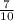`(7)/(10)` = 0.7, which equals 70%

The probability that a particular student does not walk to school is
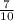`(7)/(10)` = 0.7, which equals 70%.

d. Number of favorable cases= number of students that do not walk or come by car= 320 students - number of students that walk to school - number of students that arrive by car= 320 students - 96 students - 16 students= 208 students

So:
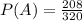
`P(A)=(208)/(320)`

P(A)=
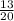`(13)/(20)` = 0.65, which equals 65%

The probability that a particular student does not walk or come by car is
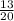`(13)/(20)` = 0.65, which equals 65%.