Question

Air expands through a turbine from 8 bar, 960 K to 1 bar, 450 K. The inlet velocity is small compared to the exit velocity of 90 m/s. The turbine operates at steady state and develops a power output of 2500 kW. Heat transfer between the turbine and its surroundings and potential energy effects are negligible. Modeling air as an ideal gas, calculate the mass flow rate of air and the exit area.

Answer 1

This question is incomplete, the missing diagram is uploaded along this answer below.

Answer:

- The mass flow rate of air is 4.59 kg/s

- Area of the exit turbine is 0.066 m²

Step-by-step explanation:

Given the data in the question;

Using air as an ideal gas.

Turbine;

state 1: p₁ = 8 bar, T₁ = 960 K

state 2: p₂ = 1 bar, T₂ = 450 K

The inlet velocity is small compared to the exit velocity of 90 m/s

V₁ < V₂ = 90 m/s

Power of turbine W' = 2500 kW

Now, lets assume Q = 0 and ΔPE = 0

we know that; R = 0.2870 kJ/kg-K

Also.
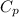`C_p` at 700K = 1.075 kJ/kg-K

Now, using ideal gas law;

STATE1

v₁ = RT₁/p₁

so we substitute

v₁ = [(0.2870 kJ/kg-K)(960 K)/(8 bars )]|
`(bar)/(10^5N/m^2)`||
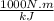`(1000N.m)/(kJ)`| = 0.344 m³/kg

STATE2

v₂ = RT₂/p₁

we substitute

v₂ = [(0.2870 kJ/kg-K)(450 K)/(1 bars )]|
`(bar)/(10^5N/m^2)`||
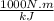`(1000N.m)/(kJ)`| = 1.2915 m³/kg

Now, from energy balance steady state;

0 = Q" - W" +
`m`(
`h_i` +
`(V_i^2)/(2)` +
`gz_i`) -
`m`(
`h_B` +
`(V_B^2)/(2)` +
`gz_B`)

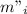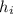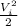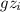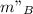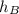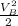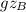since we initially assume Q = 0 and ΔPE = 0

hence;

0 =
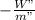`-(W` + h₁ +
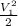`(V_1^2)/(2)` - h₂ +
`(V_2^2)/(2)`

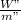`(W` = h₁ +
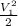`(V_1^2)/(2)` - h₂ +
`(V_2^2)/(2)`

⇒
`(W` =
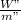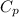`C_p`(T₁ - T₂) +
`(1)/(2)`( V₁² - V₂²)

we solve for m"

m" = W" / (
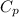`C_p`(T₁ - T₂) +
`(1)/(2)`( V₁² - V₂²))

so we substitute;

m" = {[2500 kW] / [(1.075kJ/kg-K(960K - 450k) +
`(1)/(2)`( (0)² - (90 m/s)²)) |kJ/1000N.m| |N/kg.m/s²|]} | kJ/s / kW|

m" = 4.59 kg/s

Therefore, the mass flow rate of air is 4.59 kg/s

From the mass body steady state;

0 = m"₁ - m"₂

so

m"₁ = m"₂ = 4.59 kg/s

now, the volumetric flow rate V"₂ will be;

V"₂ = m" × v₂

we substitute

V"₂ = 4.59 kg/s × 1.2915 m³/kg = 5.93 m³/s

and

the volumetric flow rate V"₂ = A₂V₂

so;

Exit Area A = V"₂ / V₂

we substitute

A = (5.93 m³/s ) / (90 m/s)

A = 0.06589 ≈ 0.066 m²

Therefore, Area of the exit turbine is 0.066 m²