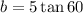Answer:
See Explanation
Explanation:
The question is incomplete as the image that illustrates the scenario is not given.
However, I can deduce that the question is about a right-angled triangle.
So, I will give a general explanation on how to find each of the side of the triangle, given a side and an angle.
For triangle A (solve for b)
Using cosine formula.
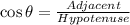
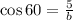
Make b the subject
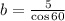
For triangle B (solve for b)
Using cosine formula.
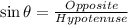
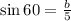
Make b the subject

For triangle C (solve for b)
Using cosine formula.
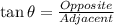

Make b the subject