Answer:
We have to flip the coin 78 times.
Explanation:
In a sample with a number n of people surveyed with a probability of a success of
 , and a confidence level of
, and a confidence level of
 , we have the following confidence interval of proportions.
, we have the following confidence interval of proportions.

In which
z is the zscore that has a pvalue of
 .
.
The margin of error is:

We suspect that the coin is fair.
This means that
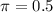
96.5% confidence level
So
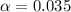 , z is the value of Z that has a pvalue of
, z is the value of Z that has a pvalue of
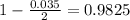 , so
, so
 .
.
How many times would we have to flip the coin in order to obtain a 96.5% confidence interval of width of at most .12 for the probability of flipping a head?
n times, and n is found when M = 0.12. So

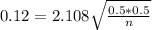
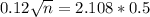

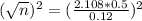

Rounding up
We have to flip the coin 78 times.