Question

Brenya Estate produces a high quality tea branded A, B and C in the ration 1.5: 5: 1. Originally Type A tea costs 1600, type B costs 800 and type C costs 1700 per kg to produce. Brenya Tea Estate packs Super tea in packets of 825g each. Blending and packing costs are 40 per kg. Determine the production cost for a packet of super tea

Answer 1

The production cost for a packet of super tea is 924.

Explanation:

The production cost of the Super tea is given as

`Cost_(super\ tea)=Cost_(A)+Cost_(B)+Cost_(C)+Cost_(packaging\ and\ blending)`

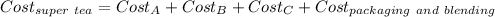
Here the costs are given as following

`Cost_A=(Ratio\ of\ A)/(Sum\ of \ ratios) * Weight * Cost\ of\ A\ per\ kg`

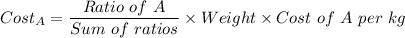
Here

• Ratio of A is 1.5
• Sum of Ratios is 1.5+5+1=7.5
• Weight is 825 g or 0.825kg
• Cost of A per kg is 1600

So

`Cost_A=(Ratio\ of\ A)/(Sum\ of \ ratios) * Weight * Cost\ of\ A\ per\ kg\\Cost_A=(1.5)/(7.5) * 0.825 * 1600\\Cost_A=264`

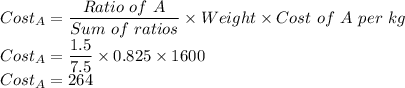
The cost of A is 264.

Similarly

`Cost_B=(Ratio\ of\ B)/(Sum\ of \ ratios) * Weight * Cost\ of\ B\ per\ kg`

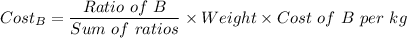
Here

• Ratio of B is 5
• Sum of Ratios is 7.5
• Weight is 825 g or 0.825kg
• Cost of B per kg is 800

`Cost_B=(Ratio\ of\ B)/(Sum\ of \ ratios) * Weight * Cost\ of\ B\ per\ kg\\Cost_B=(5)/(7.5) * 0.825 * 800\\Cost_B=440`

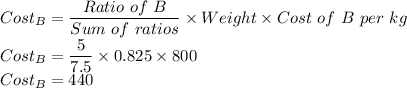
The cost of B is 440.

Also

`Cost_C=(Ratio\ of\ C)/(Sum\ of \ ratios) * Weight * Cost\ of\ C\ per\ kg`

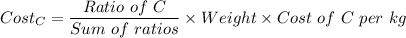
Here

• Ratio of C is 1
• Sum of Ratios is 7.5
• Weight is 825 g or 0.825kg
• Cost of C per kg is 1700

`Cost_C=(Ratio\ of\ C)/(Sum\ of \ ratios) * Weight * Cost\ of\ C\ per\ kg\\Cost_C=(1)/(7.5) * 0.825 * 1700\\Cost_C=187`

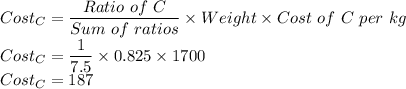
The cost of C is 187.

Now the packaging and blending cost for the given package is calculated as

`Cost_(packaging\ and\ blending)=Weight*Cost_(packaging\ and\ blending\ per\ kg)`

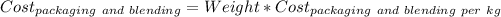
Here

• Weight is 825 g or 0.825kg
• Cost of packaging and blending per kg is 40

`Cost_(packaging\ and\ blending)=Weight*Cost_(packaging\ and\ blending\ per\ kg)\\Cost_(packaging\ and\ blending)=0.825*40\\Cost_(packaging\ and\ blending)=33`

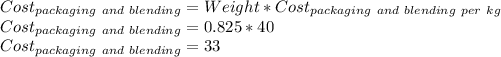
Cost of packaging and blending per packet is 33.

So now substituting values of costs in the following equation gives:

`Cost_(super\ tea)=Cost_(A)+Cost_(B)+Cost_(C)+Cost_(packaging\ and\ blending)\\Cost_(super\ tea)=264+440+187+33\\Cost_(super\ tea)=924`

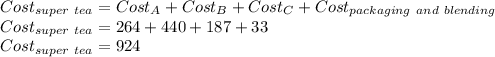
The production cost for a packet of super tea is 924.