Answer:
F = 520 N
Step-by-step explanation:
For this exercise the rotational equilibrium equation should be used
Σ τ = 0
Let's set a reference system with the origin at the back of the refrigerator and the counterclockwise rotation as positive. On the x-axis it is horizontal directed outward, eg the horizontal y-axis directed to the side and the z-axis vertical
Torque is
τ = F x r
the bold indicate vectors, we analyze each force
the applied force is horizontal along the -x axis, the arm (perpendicular distance) is directed in the z axis,
The weight of the body is the vertical direction of the z-axis, so the arm is on the x-axis
-F z + W x = 0
F z = W x
F =
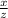 W
W
The exercise indicates the point of application of the force z = 1.5 m and the weight is placed in the center of mass of the body x = 0.6 m, we are assuming that the force is applied in the wide center of the refrigerator
let's calculate
F = 1300 0.6 / 1.5
F = 520 N