Answer:
She needs to sample 189 trees.
Explanation:
We have that to find our
 level, that is the subtraction of 1 by the confidence interval divided by 2. So:
level, that is the subtraction of 1 by the confidence interval divided by 2. So:
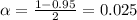
Now, we have to find z in the Ztable as such z has a pvalue of
 .
.
That is z with a pvalue of
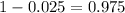 , so Z = 1.96.
, so Z = 1.96.
Now, find the margin of error M as such
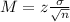
In which
 is the standard deviation of the population and n is the size of the sample.
is the standard deviation of the population and n is the size of the sample.
The standard deviation of the population is 70 peaches per tree.
This means that
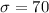
How many trees does she need to sample to obtain an average accurate to within 10 peaches per tree?
She needs to sample n trees.
n is found when M = 10. So
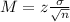
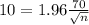
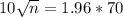
Dividing both sides by 10:
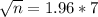

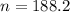
Rounding up:
She needs to sample 189 trees.