Question

5) Crystal wants to consolidate her bills into 1 smaller monthly payment. She has a total of

$23,000 worth of debt. If she consolidates with a personal loan, she can choose between a 5
year or 8 year loan at 6.75% APR. How much more interest will she pay if she chooses 8
years instead of the 5 year loan?​

Answer 1

The amount more in interest she will pay if she choose 8 years instead of 5 years loan is approximately $2,665.92

Explanation:

The total worth of debt Crystal has. P = $23,000

The options on the number of years for which the loans for consolidation are available, t = 5 and 8 years

n = 12 × t = The number of monthly payment = 12×5 = 60 or 12 × 8 = 96

The annual percentage rate of the loans, r = 6.75% = 0.0675

The monthly payments, 'M', Crystal pays is given as follows;

`M = (P \cdot \left((r)/(12) \right )\cdot \left ( 1 + (r)/(12) \right)^(n) )/(\left ( 1 + (r)/(12) \right)^(n) - 1)`

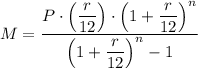
When n = 60, we get;

`M = (23,000 * \left((0.0675)/(12) \right )\cdot \left ( 1 + (0.0675)/(12) \right)^(60) )/(\left ( 1 + (0.0675)/(12) \right)^(60) - 1) \approx 452.72`

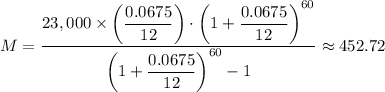
The total amount payed, A = M × n

∴ The total amount payed when n = 60 is, A₅ = 452.72 × 60 = 27163.2

A₅ ≈ $ 27,163.2

The interest on the loan, I₅ = A₅ - P

∴ I₅ = $27,163.2 - $23,000 = $4,163.2

When n = 80, we get;

`M = (23,000 * \left((0.0675)/(12) \right )\cdot \left ( 1 + (0.0675)/(12) \right)^(96) )/(\left ( 1 + (0.0675)/(12) \right)^(96) - 1) \approx 310.72`

∴ The total amount payed when n = 80 is, A₈ = 310.72 × 96 = 29,829.12

A₈ = $ 29,829.12

The interest on the loan, I₈ = A₈ - P

∴ The interest on the loan, I₈ = 29,829.12 - 23,000 = 6,829.12

The interest Crystal pays when takes the loan for 8 years, I₈ = $6,829.12

Therefore, the amount more interest she will pay if she choose 8 years instead of 5 years loan, ΔI = I₈ - I₅

ΔI = $6,829.12 - $4,163.2 = $2,665.92

The amount more interest she pays if she choose 8 years instead of 5 years loan, ΔI = $2,665.92.