Answer:

Explanation:
We are given the equation:
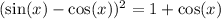
And we want to find all solutions for the equation within the interval [0, 2π).
First, we can expand. This yields:
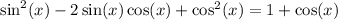
From the Pythagorean Identity, we know that:
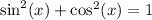
Therefore:
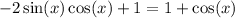
Simplify:
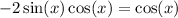
Subtract cos(x) from both sides*:

Factor:

Zero Product Property:
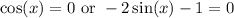
Solve for each case:
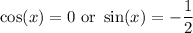
Use the unit circle. So, our solutions are:

*Note that we should not simply divide both sides by cos(x) to acquire -2sin(x) = 1. This is because we do not know what the value of x is, and so one or may values of x may result in cos(x) = 0, and we cannot divide by 0. Hence, we need to subtract and factor and utilize the Zero Product Property.