Question

What is the value of x, rounded to the nearest tenth?

Answer 1

20.8

Explanation:

`24 = \sqrt{ {12}^(2) + {x}^(2) }`

`{24}^(2) = 144 + {x}^(2)`

`576 - 144 = {x}^(2)`

`{x}^(2) = 432`

x=20.8

Answer 2

x ≈ 31.8

Explanation:

Using Pythagoras' identity in the right triangle.

The square on the hypotenuse is equal to the sum of the squares on the other 2 sides, that is

x² + 12² = 34²

x² + 144 = 1156 ( subtract 144 from both sides )

x² = 1012 ( take square root of both sides )

x =
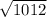`√(1012)` ≈ 31.8 ( to the nearest tenth )