Question

Find the measure of the (2y-1)° angle

Answer 1

69 degrees

Explanation:

1) Find x

The angles measured x degrees and (x-28) degrees have a sum of 180 degrees because straight lines always have a measure of 180 degrees. Knowing this, construct the equation:

`x+x-28=180\\2x-28=180`

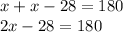
Add 28 to both sides

`2x-28+28=180+28\\2x=208`

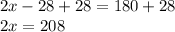
Divide both sides by 2

`(2x)/(2)= (208)/(2) \\x= 104`

Therefore, x is equal to 104 degrees.

2) Find the measure of the (x-28) degree angle

Plug x into x-28

`x-28\\= 104-28\\= 76`

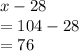
Therefore, the measure of this angle is 76 degrees.

3) Find y

All the interior angles in any triangle will add up to 180 degrees. Knowing this, we can construct another equation:

`(2y-1)+76+y= 180`

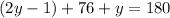
Open up the parentheses

`2y-1+76+y= 180\\3y+75= 180`

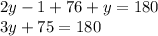
Subtract both sides by 75

`3y+75-75=180-75\\3y=105`

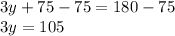
Divide both sides by 3

`(3y)/(3)= (105)/(3) \\y=35`

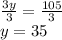
Therefore, y is equal to 35 degrees.

4) Find the measure of the (2y-1) degree angle

Plug y into 2y-1

`2y-1\\= 2(35)=1\\= 70-1\\= 69`

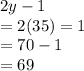
Therefore, y is equal to 69 degrees.

I hope this helps!