Question

PLEASE HELP ASAP

P(-5, 2), Q(4, 5), R(6, – 1), S(-3, -4)
this is a rectangle right and why is it and not rhombus or square?

Answer 1

It's a rectangle, but not a rhombus (or square).

Explanation:

Let's see the vectors of each next vertex:

`Q - P = (4, 5) - (-5, 2) = (9, 3)\\R - Q = (6, -1) - (4, 5) = (2, -6)\\S - R = (-3, -4) - (6, -1) = (-9, -3)\\P - S = (-5, 2) - (-3, -4) = (-2, 6)\\`

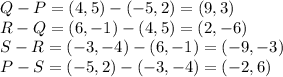
Firstly, we can notice that it's a parallelogram - because the Q-P side is parallel to the S - R side (if the x:y ratios are the same, the sides are parallel).

A rhombus needs the sides to be of the same length. But the length of a (x, y) vector is
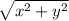`√(x^2 + y^2)` and
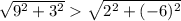`√(9^2 + 3^2) > √(2^2 + (-6)^2)`, we don't even have to compute it exactly. If it's not a rhombus, it's also not a square (every square is a rhombus).

The last thing left is to know if it's a rectangle - for it to be a rectangle, we need to check if the vectors are perpendicular.

We can compute the dot product of the vectors - perpendicular vectors always have a dot product equal to zero. The dot product of two vectors
`(x_1, y_1)` and
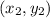`(x_2, y_2)` is equal to
`x_1x_2 + y_1y_2`.

`(9, 3) \cdot (2, -6) = 9\cdot 2 + 3\cdot(-6) = 18 - 18 = 0`

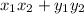So the sides are perpendicular, and as such - the figure is a rectangle.