Answer :
Here, It is stated that the triangle having sides 3, 5 and 6 respectively. So, we have to prove that whether the given triangle is right angled triangle or not.
For That :
We will be using the Pythagoras Theorem
Given Sides :-
According to the Pythagoras Theorem , the sum of the square of Base side and perpendicular side is equal to the square of the longest side i.e, hypotenuse.
Pythagoras Theorem :
⠀⠀⠀⠀⠀⠀

So, here the longest side is Side A of measurement 6.
So, Now
Applying Pythagoras Theorem in it, we get :
The three sides , so let's plug the values in the formula :-
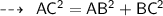
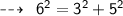
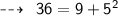
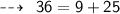
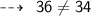
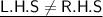
If in a triangle, the square of the hypotenuse is not equal to the sum of the squares of the other two sides, then the given triangle and doesn't satisfy pythagoras theorem. Hence, Its not a right angled triangle.