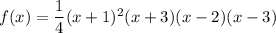Answer:
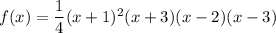
Tia is correct
Explanation:
From inspection of the graph, we can say that the leading coefficient of the function is positive as:
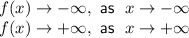
From inspection of the graph, we can see that the curve intercepts the x-axis at x = -3, x = 2, x = 3
As the curve touches the x-axis at (-1, 0) then this means that the root x = -1 has multiplicity 2
Therefore,
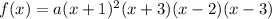 where
where
 is some positive constant.
is some positive constant.
If we multiply the constants, we can determine the y-intercept:
y-intercept = a x 1² x 3 x -2 x -3 = 18a
Therefore, the y-intercept is at y = 18a
From inspection of the graph, the y-intercept is at y = 4.5
So to determine the value of a: 18a = 4.5 ⇒ a = 1/4
Therefore, the fully factored form of the equation is: