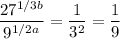Question

A and b are positive integers and a-b=2. Evaluate the following:

(27^1/3b)/ ( 9^1/2a)

(this is urgent)

(I have tried 1, 25, and 1/27. None of them are correct)

Answer 1

`\displaystyle\displaystyle (27^(1/3b))/(9^(1/2a))=(1)/(9)`

Explanation:

We are given that a and b are positive integers such that:

`a-b=2`

And we want to evaluate:

`\displaystyle (27^(1/3b))/(9^(1/2a))`

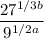
First, note that 27 = 3³ and that 9 = 3². Therefore:

`\displaystyle =((3^3)^(1/3b))/((3^2)^(1/2a))`

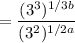
Simplify:

`=\displaystyle (3^b)/(3^a)`

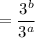
Using the quotient property of exponents:

`=3^(b-a)`

From our given equation, we can divide both sides by -1 to acquire:

`-a+b=-2\text{ or } b-a=-2`

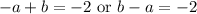
Therefore:

`=3^(-2)`

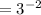
Hence, our answer is:

`\displaystyle\displaystyle (27^(1/3b))/(9^(1/2a)) =(1)/(3^2)=(1)/(9)`