Answer:
72.57° North of east
Step-by-step explanation:
From the given information:
We can compute the velocity plane that is related to the ground in air in the North direction as;
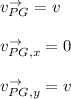
However, the velocity of the wind-related to the ground from the NorthEast direction is;

Now,
Since the plane is moving with a 236 km/h speed in the Northeast direction;
Then;
