Question

Questions (a,b), 1 (a,b)

Answer 1

a )

First of all we need to find the value of x ,

because the angles are written in terms of the variable x .

______________________________________

Let's find the value of x :

STV angle & SUV angle have same measure because both of them are the front angle of SV arc .

`STV angle \: = SUV angle \: \: = (SV \: arc)/(2) \\`

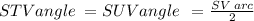
So :

`3x - 5 = 2x + 15`

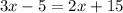
Add sides 5

`3x - 5 + 5 = 2x + 15 + 5`

`3x = 2x + 20`

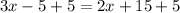
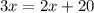
Subtract sides minus 2x

`3x - 2x = 2x + 20 - 2x`

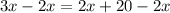
Collect like terms

`x = 2x - 2x + 20`

`x = 20`

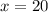
Thus the measure of angle T equals :

`measure \: of \: angle \: T = 3x - 5 \\`

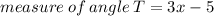
Now just need to put the value of x which we found :

`measure \: of \: angle \: T \: = 3 * (20) - 5 \\`

`measure \: of \: angle \: T \: = 60 - 5`

`measure \: of \: angle \: T \: = 55°`

♡♡♡♡♡♡♡♡♡♡♡♡♡♡♡♡♡♡♡♡♡♡

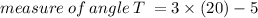
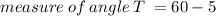
b )

angle S & angle V are also have same measure because they both are the front angles to the TU arc .

And we need to find the value of x again in this part exactly like we did for a .

`angle \: \: S = angle \: \: V`

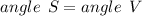
As the question told :

`angle \: \: S = 3x`

and ,

`angle \: \: V = x + 16`

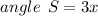
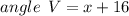
Thus :

`3x = x + 16`

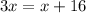
Subtract sides minus x

`3x - x = x + 16 - x`

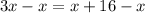
Collect like terms

`2x = x - x + 16`

`2x = 16`

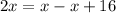
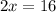
Divide sides by 2

`(2x)/(2) = (16)/(2) \\`

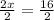
Simplification

`x = 8`

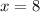
So ;

`measure \: \: of \: \: angle \: \: S = 3x`

`measure \: \: of \: \: angle \: \: S = 3(8)`

`measure \: \: of \: \: angle \: \: S = 24°`

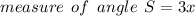
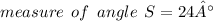
And we're done.