Question

Which of the given numbers is not a Pythagorean triplets. *

1 point

8,15,17

9,40,41

4,7,8​

Answer 1

Explanation:

By Pythagoras' Theorem,

`{c}^(2) = {a}^(2) + {b}^(2)`

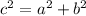
where c is always the largest number.

a and b can be interchangeable between the 2nd largest and the 3rd largest numbers.

Given a = 8, b = 15 and c = 17,

`{a}^(2) + b {}^(2) = {8}^(2) + {15}^(2) \\ = 64 + 225 \\ = 289 \\ \\ {c}^(2) = {17}^(2) \\ = 289`

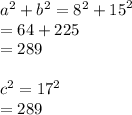
Since c^2 = a^2 + b^2 , 8 , 15 and 17 are pythagorean triplets.

Now let's move on to 9, 40 and 41.

`{a}^(2) + {b}^(2) = {9}^(2) + {40}^(2) \\ = 81 + 1600 \\ = 1681 \\ \\ {c}^(2) = {41}^(2) \\ = 1681`

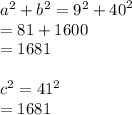
Since c^2 = a^2 + b^2 , 9 , 40 and 41 are pythagorean triplets.

Last let's move on to 4,7 and 8.

`{a}^(2) + {b}^(2) = {4}^(2) + {7}^(2) \\ = 16 + 49 \\ = 65 \\ \\ {c}^(2) = {8}^(2) \\ = 64`

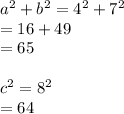
Since a^2+b^2 IS NOT EQUAL to c^2, 4,7 and 8 ARE NOT pythagorean triplets.