Question

Solving Rational Functions Hello I'm posting again because I really need help on this any help is appreciated!!​

Answer 1

x = √17 and x = -√17

Explanation:

We have the equation:

`(3)/(x + 4) - (1)/(x + 3) = (x + 9)/((x^2 + 7x + 12))`

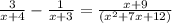
To solve this we need to remove the denominators.

Then we can first multiply both sides by (x + 4) to get:

`(3*(x + 4))/(x + 4) - ((x + 4))/(x + 3) = ((x + 9)*(x + 4))/((x^2 + 7x + 12))`

`3 - ((x + 4))/(x + 3) = ((x + 9)*(x + 4))/((x^2 + 7x + 12))`

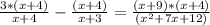
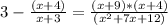
Now we can multiply both sides by (x + 3)

`3*(x + 3) - ((x + 4)*(x+3))/(x + 3) = ((x + 9)*(x + 4)*(x+3))/((x^2 + 7x + 12))`

`3*(x + 3) - (x + 4) = ((x + 9)*(x + 4)*(x+3))/((x^2 + 7x + 12))`

`(2*x + 5) = ((x + 9)*(x + 4)*(x+3))/((x^2 + 7x + 12))`

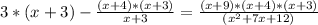
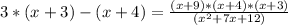
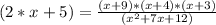
Now we can multiply both sides by (x^2 + 7*x + 12)

`(2*x + 5)*(x^2 + 7x + 12) = ((x + 9)*(x + 4)*(x+3))/((x^2 + 7x + 12))*(x^2 + 7x + 12)`

`(2*x + 5)*(x^2 + 7x + 12) = (x + 9)*(x + 4)*(x+3)`

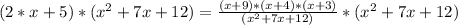
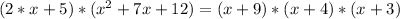
Now we need to solve this:

we will get

`2*x^3 + 19*x^2 + 59*x + 60 = (x^2 + 13*x + 3)*(x + 3)`

`2*x^3 + 19*x^2 + 59*x + 60 = x^3 + 16*x^2 + 42*x + 9`

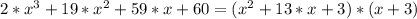
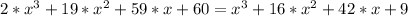
Then we get:

`2*x^3 + 19*x^2 + 59*x + 60 - ( x^3 + 16*x^2 + 42*x + 9) = 0`

`x^3 + 3x^2 + 17*x + 51 = 0`

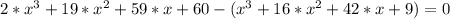
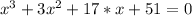
So now we only need to solve this.

We can see that the constant is 51.

Then one root will be a factor of 51.

The factors of -51 are:

-3 and -17

Let's try -3

p( -3) = (-3)^3 + 3*(-3)^2 + +17*(-3) + 51 = 0

Then x = -3 is one solution of the equation.

But if we look at the original equation, x = -3 will lead to a zero in one denominator, then this solution can be ignored.

This means that we can take a factor (x + 3) out, so we can rewrite our equation as:

`x^3 + 3x^2 + 17*x + 51 = (x + 3)*(x^2 + 17) = 0`

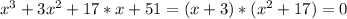
The other two solutions are when the other term is equal to zero.

Then the other two solutions are given by:

x = ±√17

And neither of these have problems in the denominators, so we can conclude that the solutions are:

x = √17 and x = -√17