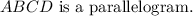Question

Heya!


`\underline{ \underline{ \text{QUESTION : }}}` In the given quadrilateral PQRS, the mid-points of the sides PQ , QR , RS and SP are A , B , C and D respectively. Prove that ABCD is a parallelogram.

Answer 1

this is your answer look it once

Answer 2

See Below.

Explanation:

We are given that A, B, C, and D are the midpoints of sides PQ, QR, RS, and SP, respectively.

And we want to prove that ABCD is a parallelogram.

By the definition of midpoint, this means that:

`SD\cong DP, \, PA\cong AQ, \, QB\congBR, \, \text{ and } RC\cong CS`

To prove, we can construct a segment from S to Q to form SQ. This is shown in the first diagram.

By the Midpoint Theorem:

`DA\parallel SQ`

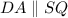
Similarly:

`CB\parallel SQ`

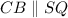
By the transitive property for parallel lines:

`DA\parallel CB`

Likewise, we can do the same for the other pair of sides. We will construct a segment from P to R to form PR. This is shown in the second diagram.

By the Midpoint Theorem:

`AB\parallel PR`

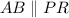
Similarly:

`DC\parallel PR`

So:

`AB\parallel DC`

This yields:

`DA\parallel CB\text{ and } DC\parallel AB`

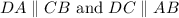
By the definition of a parallelogram, it follows that:

`ABCD\text{ is a parallelogram.}`