Question

Which is not a correct way to rewrite this expression using the distributive

property?
(2x2 + 4x - 7)(x - 2)

A. (2x2 + 4 – 7)(x) + (2x2 + 4x - 7)(x - 2)
B. (2x2)(x) + (2x2)(-2) + (4x)(x) + (4x)(-2) + (-7)(x) + (-7)(-2)
C. (2x2)(x - 2) + (4x)(x - 2) + (-7)(x - 2)
D. (2x2 + 4x - 7)(x) + (2x2 + 4x - 7)(-2)

Answer 1

Given:

The expression is

`(2x^2+4x-7)(x-2)`

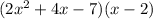
To find:

The expression which is not a correct way to rewrite the given expression.

Solution:

We have,

`(2x^2+4x-7)(x-2)`

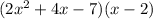
Using distributive property, we get

`=(2x^2)(x-2)+(4x)(x-2)+(-7)(x-2)`

`=(2x^2)(x)+(2x^2)(-2)+(4x)(x)+(4x)(-2)+(-7)(x)+(-7)(-2)`

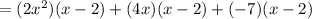
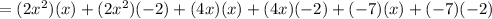
Using distributive property the given expression can rewritten as:

`=(2x^2+4x-7)(x)+(2x^2+4x-7)(-2)`

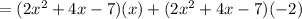
Only the expression in option A is not a correct way to rewrite the given expression because
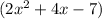`(2x^2+4x-7)` is not distributed to
`(x-2)` properly.

Therefore, the correct option is A.