Question

For each pair of functions f and g below, find f(g(x)) and g(f(x)).

Then, determine whether f and g are inverses of each other.
Simplify your answers as much as possible.
(Assume that your expressions are defined for all x in the domain ofthe composition.
You do not have to indicate the domain.)

Answer 1

a) f(g(x)) =
`(1)/(2((1)/(2x) ))` =
`(1)/((1)/(x) ) }` = x

g(f(x)) =
`(1)/(2((1)/(2x) ))` =
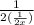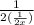`(1)/((1)/(x) ) }` = x

f and g are inverses

b) f(g(x)) = x + 3 + 3 = x + 6

g(f(x)) = x + 3 + 3 = x + 6

f and g are not inverses

Explanation:

a)

f(g(x)) =
`(1)/(2((1)/(2x) ))` =
`(1)/((1)/(x) ) }` = x

g(f(x)) =
`(1)/(2((1)/(2x) ))` =
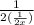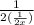`(1)/((1)/(x) ) }` = x

Since f(g(x)) = g(f(x)) = x, then f and g are inverses

b)

f(g(x)) = x + 3 + 3 = x + 6

g(f(x)) = x + 3 + 3 = x + 6

Since f(g(x)) = g(f(x)) ≠ x, then f and g are not inverses