Answer:
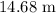
Masses of the truck and load
Step-by-step explanation:
v = Final velocity = 0
u = Initial velocity = 12 m/s
 = Coefficient of friction = 0.5
= Coefficient of friction = 0.5
a = Acceleration
g = Acceleration due to gravity =
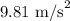
s = Displacement
f = Friction
F = Force applied
The force balance of the system is given by
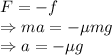
From the kinematic equations we have
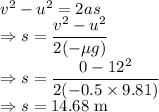
The minimum stopping distance for which the load will not slide forward relative to the truck is
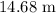
As it can be seen that the masses of the truck and load are not used in the above used formulae. So, they are unnecessary.