Question

A power cycle operates between hot and cold reservoirs at 600 K and 300 K, respectively. At steady state the cycle develops a power output of 0.45 MW while receiving energy by heat transfer from the hot reservoir at the rate of 1 MW. a. Determine the thermal efficiency and the rate at which energy is rejected by heat transfer to the cold reservoir, in MW. b. Compare the results of part (a) with those of a reversible power cycle operating between these reservoirs and receiving the same rate of heat transfer from the hot reservoir

Answer 1

a. The thermal efficiency of the actual cycle is 45%

ii) The rate at which energy is rejected by heat transfer to the cold reservoir is 0.55 MW

b. The (maximum) cycle efficiency, is 50%

The rate of energy rejection to the cold reservoir by heat transfer is 0.55 MW

Therefore, there is an increased efficiency and reduced heat rejection in the reversible power cycle working between the given two reservoirs

Step-by-step explanation:

The given parameters are;

The temperature of the hot reservoir,
`T_H` = 600 K

The temperature of the hot reservoir,
`T_C` = 300 K

The power output,
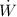`\dot W` = 0.45 MW = The cycle work per second

The heat input from the hot reservoir,
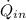`\dot Q_(in)` = 1 MW

a. The thermal efficiency of the actual cycle is given by the work done divided by the heat supplied as follows;

`\eta_(actual) = (\dot W)/(\dot Q_(in))`

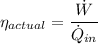
Therefore, we have;

`\eta_(actual) = (0.45 \ MW)/(1 \ MW) = 0.45 = 45 \%`

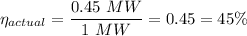
The thermal efficiency of the actual cycle,
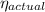`\eta_(actual)` = 45%

ii) The rate at which energy is rejected by heat transfer to the cold reservoir,
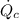`\dot Q_(c)`, is given as follows;

`\dot Q_(in)` =
`\dot Q_(c)` +
`\dot W`

∴
`\dot Q_(c)` =
`\dot Q_(in)` -
`\dot W`

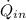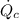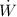
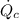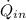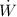
Therefore, we have;

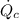`\dot Q_(c)` = 1 MW - 0.45 MW = 0.55 MW

The rate at which energy is rejected by heat transfer to the cold reservoir,
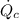`\dot Q_(c)` = 0.55 MW

b. For a reversible power cycle, we have the maximum cycle efficiency,
`\eta_(maximum)`, given as follows;

`\eta_(maximum) = (T_H - T_C)/(T_H)`

`\therefore \eta_(maximum) = (600 \, K - 300 \, K)/(600 \, K) = 0.5 = 50\%`

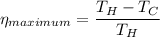
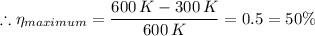
The maximum cycle efficiency,
`\eta_(maximum)` = 50%

The rate of work done by the cycle,
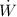`\dot W`, is therefore give as follows;

`\eta_(maximum) = (\dot W)/(\dot Q_(in))`

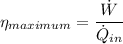
Therefore;

`\dot Q_(in)` ×
`\eta_(maximum)` =
`\dot W`

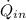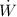
1 MW × 50% = 0.5 MW =
`\dot W`

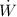
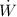`\dot W` = 0.5 MW

`\dot Q_(c)` =
`\dot Q_(in)` -
`\dot W`

∴
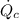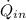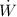
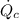`\dot Q_(c)` = 1 MW - 0.5MW = 0.5MW

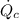`\dot Q_(c)` = 0.5MW

The rate of energy rejection to the cold reservoir by heat transfer,
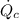`\dot Q_(c)` = 0.55 MW

Therefore the frequency and the rate at which energy is rejected by heat transfer to the cold reservoir are both higher for the reversible power cycle