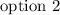Question

What is the solution set of 2x2 - 3x = 5?

1.) -1,-5/2
2.)-1,5/2
3.) 1,-5/2

i really need this to be answer correctly, the test grade is high

Answer 1

2.)-1,5/2 is your answer

Explanation:

2x2 - 3x = 5

2x²-3x-5=0

do ing middle term factorization

2x²-5x+2x-5=0

x(2x-5)+1(2x-5)=0

(2x-5)(x+1)=0

either

x=5/2

or

x=-1

Answer 2

`\displaystyle \: 2) - 1,(5)/(2)`

Explanation:

we are given a quadratic equation

`\displaystyle \: {2x}^(2) - 3x = 5`

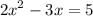
we have to determine the roots

to do so first bring it to standard form i.e

ax²+bx+c=0

move left hand side expression to right hand side and change its sign:

`\displaystyle \: {2x}^(2) - 3x - 5 =0`

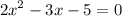
since we moved left hand side expression to right hand side there's only 0 left to the left hand side

we'll use factoring method to solve the quadratic

first, we need to express the middle term as a sum or subtraction of two different terms

to do so

rewrite -3x as 2x-5x:

`\displaystyle \: {2x}^(2) + 2x - 5x- 5 =0`

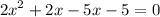
now we have two common factors 2x and -5 so

factor them out:

`\displaystyle \: 2x ({x} + 1 )- 5(x + 1) =0`

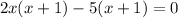
group:

`\displaystyle \: (2x - 5) (x + 1) =0`

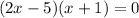
since 2x-5 and x+1 both equal 0

separate the equation as two different equation:

`\displaystyle \: \begin{cases}2x - 5=0 \\ x + 1 = 0\end{cases}`

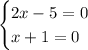
let's work with the first equation:

add 5 to both sides:

`\displaystyle \: 2x - 5 + 5 = 0 + 5 \\ 2x = 5`

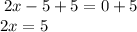
divide both sides by 2:

`\displaystyle \: (2x)/(2) = (5)/(2) \\ \therefore \: x_{} = (5)/(2)`

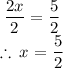
let's work with the second one

cancel 1 from both sides:

`\displaystyle \: x + 1 - 1 = 1 - 1 \\ \therefore \: x = - 1`

hence,

our answer choice is
`\text{option 2}`