Answer:
The area of the triangle is 18 square units.
Explanation:
First, we determine the lengths of segments AB, BC and AC by Pythagorean Theorem:
AB
![AB = \sqrt{(5-2)^(2)+[6-(-1)]^(2)}](https://img.qammunity.org/2022/formulas/mathematics/high-school/jdjq42l4u3almgmo603ja8xfj8v54xzurx.png)
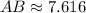
BC

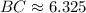
AC
![AC = \sqrt{(-1-2)^(2)+[4-(-1)]^(2)}](https://img.qammunity.org/2022/formulas/mathematics/high-school/z5w8mm8mv9lnmmzoidown9433wshbdiuxq.png)
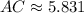
Now we determine the area of the triangle by Heron's formula:
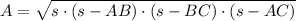 (1)
(1)
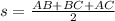 (2)
(2)
Where:
 - Area of the triangle.
- Area of the triangle.
 - Semiparameter.
- Semiparameter.
If we know that
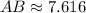 ,
,
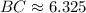 and
and
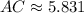 , then the area of the triangle is:
, then the area of the triangle is:

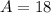
The area of the triangle is 18 square units.