Question

A manufacturer of a new nicotine nasal spray claims that their product has a 30% success rate for smoking cessation. In a clinical study involving 150 smokers, 93 of them quit smoking. Test the hypothesis that the success rate claimed by the manufacturer is valid at the 5% level of significance.

Answer 1

we reject null and conclude that this manufacturers claim is false

Explanation:

p = 30% = 0.30

p^ = 93/150 = 0.62

we state the hypothesis

H0: p = 0.30

h1: p not equal to 0.30

we find the z test stattistics

`z=\frac{p^--p}{\sqrt{(p(1-p))/(n) } }`

`z=\frac{0.62-0.30}{\sqrt{(0.30(1-0.30))/(150) } }`

`z=\frac{0.32}{\sqrt{(0.30*0.70)/(150) } }`

`z=(0.32)/(0.03741)`

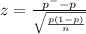
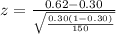
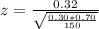
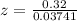
z = 8.5538

at alpha = 0.05

z-critical = Z₀.₀₅/₂ = Z₀.₀₂₅

= 1.96

we compare z critical with the test statistic

z statistic > z critical so we have to reject H₀ and conclude that the manufacturers claim is not valid at 0.05 level of significance.