Answer:
Part A
The population for the first four years are;
Year 1; 26,000,000,
Year 2; 22,100,000
Year 3; 18,785,000
Year 4; 15,967,250
Part B
i)The third year
ii) Provision of spawning environment and the reduction of predators
Part C
The population after 10 years is approximately 139,208,436
Explanation:
Part A
The given parameters are;
The rate at which the population is decreasing = 15%
The population of the walleye at the beginning of the current year, P = 26 million
Therefore, we have;
The population of subsequent year = 0.85 × The population of the current year
Therefore, we have a geometric series, with a first term, a = 26 million, and a common ratio, r = 0.85, given as follows;
aₙ = a + ar + ar² + ...
Therefore the first 4 years using 26 million as the first term are;
a₁ = 26 × 10⁶
a₂ = 26 × 10⁶ × 0.85 = 22,100,000
a₃ = 26 × 10⁶ × 0.85² = 18,785,000
a₄ = 26 × 10⁶ × 0.85³ = 15,967,250
The population for the first four years are;
26,000,000, 22,100,000, 18,785,000, 15,967,250
Part B
i) Given that the walleye population are considered to be in rehabilitation state when they are between 15 and 20 million, we have;
The population in the third year = 18,750,000 < 20,000,000
Therefore, the population enters rehabilitation state in the 3rd year
ii) Other intervention method includes provision of a adequate environment for spawning and the removal of predators
Part C
The formula for the sum of the geometric progression is presented as follows;
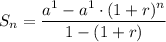
Therefore, we have;

The population after 10 years, Sₙ = 139.208436581 × 10⁶ ≈ 139,208,436