Answer:

General Formulas and Concepts:
Pre-Algebra
Order of Operations: BPEMDAS
- Brackets
- Parenthesis
- Exponents
- Multiplication
- Division
- Addition
- Subtraction
Equality Properties
- Multiplication Property of Equality
- Division Property of Equality
- Addition Property of Equality
- Subtraction Property of Equality
Algebra I
- Terms/Coefficients
- Functions
- Function Notation
- Graphing
- Solving systems of equations
Calculus
Area - Integrals
Integration Rule [Reverse Power Rule]:
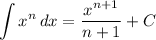
Integration Rule [Fundamental Theorem of Calculus 1]:
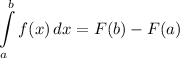
Integration Property [Addition/Subtraction]:
![\displaystyle \int {[f(x) \pm g(x)]} \, dx = \int {f(x)} \, dx \pm \int {g(x)} \, dx](https://img.qammunity.org/2022/formulas/mathematics/high-school/r5yh324r81plt97j3zrr5qi2xxczxlqi34.png)
Area of a Region Formula:
![\displaystyle A = \int\limits^b_a {[f(x) - g(x)]} \, dx](https://img.qammunity.org/2022/formulas/mathematics/college/uij08sp4x97gp23utcdwranet4linkrd6u.png)
Explanation:
*Note:
Remember that for the Area of a Region, it is top function minus bottom function.
Step 1: Define
f(x) = x²
g(x) = x⁶
Bounded (Partitioned) by x-axis
Step 2: Identify Bounds of Integration
Find where the functions intersect (x-values) to determine the bounds of integration.
Simply graph the functions to see where the functions intersect (See Graph Attachment).
Interval: [-1, 1]
Lower bound: -1
Upper Bound: 1
Step 3: Find Area of Region
Integration
- Substitute in variables [Area of a Region Formula]:
![\displaystyle A = \int\limits^1_(-1) {[x^2 - x^6]} \, dx](https://img.qammunity.org/2022/formulas/mathematics/college/s67ep4kffky0xrff9ikvib6fg0hpt6zg4l.png)
- [Area] Rewrite [Integration Property - Subtraction]:
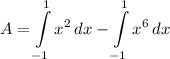
- [Area] Integrate [Integration Rule - Reverse Power Rule]:
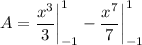
- [Area] Evaluate [Integration Rule - FTC 1]:
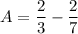
- [Area] Subtract:

Topic: AP Calculus AB/BC (Calculus I/II)
Unit: Area Under the Curve - Area of a Region (Integration)
Book: College Calculus 10e